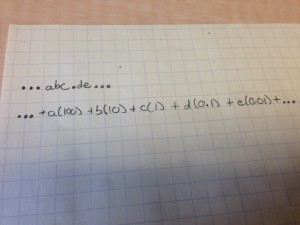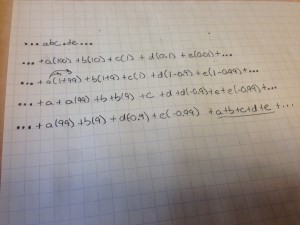So, in unusual form, I’m gonna write another blog post about a week after my last one!  I like this trend…perhaps I’ll keep it up.

I have been “Facebooked” the following video quite a bit over the last month or so.  It’s about the fantastic numerology of the circle.

While I do appreciate the sentiment, I felt compelled to share why this video is straight-up bullshit.  This will happen in three parts: the first being addressing why there actually are 360° in a circle (spoiler: it’s not anything mystical), the second being why the number 9 is not any cooler than any other number, and the third being wrap-up.

Part the First : Why are there 360º in a Circle

First off, let me say that there is no absolutely concrete answer to this…that has been lost to antiquity.  There are, however, some very compelling comments to be made.  In order to understand them, I need to tell you about the sexagesimal number system.

We are quite familiar with the decimal system, also known as base-10.  The idea behind it is pretty simple.  When you write a number with multiple digits, say 231, it is agreed that each digit place, from right to left, represents a power of the number 10.  231, for instance, can be represented as 2(100) + 3(10) + 1(1).  That’s it.  Question is, why 10?  Well, our hands…one digit for each finger (hell, we even call our fingers digits)…when we run out of fingers, we move on to the next place.

Now, the idea that we have base-10 implies that we could have base-N, where N is whatever number we want, right?  Binary, or base-2, is the most obvious example.  In this numbering system, each digit place represents a power of 2 instead of a power of 10.  We only have 2 digits (0 and 1) that we can represent a binary number with.  So, if I write 10110, that means 1(16) + 0(8) + 1(4) + 1(2) + 0(1) …consequently, the number 10110 in base-2 is equivalent to 22 in base-10.  This kind of counting is useful when the thing counting only has 2 digits; switches can only be on or off.

There are all sorts of bases that can be used for counting…the fact that we use base 10 is not amazing, it’s just anatomically convenient.  In fact, the ancient people of the Americas, such as the Incas, Mayans, and Aztecs, used something called vigesimal, which is base-20.  They did this because the counted on their fingers and their toes.

If you go way back to the Babylonians, they invented a system referred to as sexagesimal, which is base-60!  What!?  Why!?  Well, try this…hold out your hands in front of you, thumb out on your left hand and all five digits (LOL) out on your right hand.  Now, using your right thumb, point to each segment of the 4 fingers on your right hand.  Of course, assuming no wood shop accidents, you will count 12 “segments”.  When you count them all, extend the next digit on your left hand.  Do this until you extend all your left digits and you get…60!  Not so crazy after all.

Besides that, 60 is a prolific number.   It is evenly divisible by 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, and 60.  It’s quite useful for trade; if all the neighboring city-states have different currency exchange rates, use a numbering system that can accommodate all of the them.  It was used for thousands of years by the Babylonians and other cultures of ancient Mesopotamia as well as the Egyptians, who really laid the foundations for things to come.  We still use this system today, though only for measuring time and angles.

So, what about the circle?  Turns out, many of the ancient cultures used a 360-day calendar.  Why?  It comes from ancient Mesopotamian view that the sun, the moon, and the stars moved along 360-degree circuits through the sky.  It was a stellar calendar that seems to be a nice compromise between the solar year (which is about 365 days long) and the lunar year (which is about 355 days long).  So, express your cyclical calendar as a circle and you get 360 days, of 360°.

It’s easy to see how this got appropriated by mysticism.  But, it is simply an issue of practicality.

Part the Second : Revolution No. 9

Side note : the linguistic idea that the division of a thing after the primary is called “second”, such as the section title I just typed, comes from this numbering system (hour, minute, second…degree, minute, second…).

So, in this video, why is the number 9 so fantastic and prolific?  Why does every division and inscribed polygon in a circle collapse down to 9?  Turns out, it’s not remarkable at all.  Well, I suppose it is, but certainly not for any mystic reason.

You may recall a neat trick to figuring out if a number is divisible by 9: add up all the digits of a number and if the result is divisible by 9, then so is the number.  What is that all about?  Well, it’s a simple mathematical property that I will illustrate here.

Take any number at all, decimals and everything.  To illustrate this in the most general way possible, I’ll write that number like this:

where the “…” means there are as many digits as you want in either direction and the constants a, b, c, b, and e are whatever digits you want.  Now, remember what the decimal system means.  It means that we can rewrite the number like this:

 

Now, I can write each one of those numbers in the parenthesis as (1 + something), like this:

 

I can now distribute the number outside the parenthesis and then rearrange the terms to get something like this:

 

Notice the thing that I underlined…it’s the sum of all of the digits of the number I chose.  But, look at the first part.  If I factor a 9 out, I have the following:

No matter what, the first part is always divisible by 9…there’s a 9 in front of it!  It doesn’t matter what I pick for the digits.  The only thing that matters is if the second part (second LOL) is also divisible by 9. That’s the secret to the trick!  That’s why you can just add up all the digits and see if they’re divisible by 9.

Why doesn’t this work with all the numbers?  Well, it does work with 3 for the same reason; the first term is always divisible by 9, which means it’s also always divisible by 3.  So, if the second term is as well, the whole number is divisible by 3.  As for the other numbers, the key step in the process is the point where it write the numbers in parentheses as (1 + something).  It works because we use a base-10 system.  If I take any place value (1, 10, 100, 1000, so on) and subtract 1 from it, the result is always divisible by 9.  Because the number I pulled out was 1, I get a nice second term that is just the sum of the digits.  If I were to write the number in parentheses as, say, (2 + something), then that second term would have extra things in it and it wouldn’t just be as simple as adding up the digits; that’s why this only works with 3 and 9.

So, there’s no magic in it, it’s just the result of basing our counting system on the number of digits we have on our hands.

Part the Third : So what’s with the video?

The video seems magical because no matter what you do, all these digits add up to 9.  Well, there are 360° in a circle and 360 is divisible by 9.  So, if you multiply 360 by anything, the result is always divisible by 9.  No matter how you subdivide the circle, either by diameter chords (like the first part of the video) or by inscribing regular polygons (like the second part), you’ll always get a number that is divisible by 9.  Nothing to it.

As for the metaphysical meaning, well, what can I say.  Humans like to look at Nature, see patterns, and assign meaning to those patterns.  Sometimes they’re right, just as Newton’s laws.  Sometimes, they’re not, like astrology and numerology.  We are designed to pattern recognize and it’s so easy to assign meaning to nonexistent things.  The video presents a mystical link between geometry and arithmetic that seems magical, but it’s not…they’re just numbers.

The fact that we can abstract “things” in such a way as to even be able to discuss the “nine-ness” of a group of things or the fact that one can use mathematics to describe nature, that’s magic enough.  Seriously, think about the fact that I can say 9, and that it has meaning.  Primitive languages didn’t have that capacity.  They had, 1, 2, more than 2, and that was it.  The fact that we could eventually abstract number into its own concept is what made humanity the thing it is today.  None of the modern science we have would exist if it weren’t for that critical step.  That’s the mystical thing.

Let’s not make things more complicated than they have to be.